To understand Integers correctly you need to understand the rules for multiplying and dividing integers three rules:
In general math, repeated addition of the same numeric term over and over can be expressed in terms of multiplication. For example, suppose you are in a room with 4 rows of chairs, and in each row, there are 5 chairs. You could compute the total number of chairs by adding the number of chairs in each row:
5 + 5 + 5 + 5 = 20
Or you could multiply 4 times 5 (sometimes written as 4 x 5 or 4 • 5), or (4)(5) = 20
In general, if a term x occurs repeatedly n times in a sum expression, we can reduce the expression to:
n.x = x + x + x +…+ x (total n times)
1. Rules for Multiplying and Dividing Integers:
Multiplying two positive numbers
Multiply both numbers to give the result positive
In general, you learned to multiply positive numbers to obtain their result; thus, for example, 7 x 3 is 21.
Dividing two positive Integers
Dividing two positive integers yields a result positive.
Divide positive numbers to obtain their result; thus, for example, 21 / 3 is 7.
2. Rules for Multiplying and Dividing Integers: Two negative numbers
Multiplying two negative numbers
Multiply both numbers to give the result positive
Multiplying two negative numbers say -7 x -3, do the normal multiplication which is 7 x 3 = 21, and then multiply their signs. Here negative x negative number gives positive
so -7 x -3 = 21
Dividing two negative numbers
Dividing two negative integers yields a positive result.
Dividing two negative numbers say -21 / -3, do the normal division which 21 / 3 = 7, and then divide their signs. Here negative/negative number gives positive
3. Multiplying and Dividing one positive and other negative numbers
Multiplying a positive and a negative number
Multiplying a positive and a negative number give the resulting negative
Here, when you multiply positive and negative numbers say -7 x 3, do the normal multiplication which is 7 x 3 = 21, and then multiply their signs. Here negative x positive number gives negative.
So -7 x 3 = – 21
Dividing one positive and other negative number
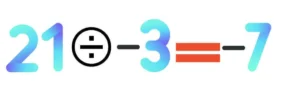
Dividing positive and negative integers yields a negative result.
So, when you divide one positive and other negative numbers say 21 / -3, do the normal division which 21 / 3 = 7 and then divide their signs. Here positive/negative number or a negative number/ positive number gives a negative.
So, 21 / -3 = -7
Conclusion:
– x + = –
+ x – = –
– x – = +
+ x + = +
