Adding and Subtracting Polynomials In Math 3 Days

Adding and Subtracting Polynomials are the operations performed on a polynomial.

Basic Requirements:

Adding and subtracting are two of the most basic operations you have. When solving equations with variables on each side, you have been able to
rearrange all variables to be on one side of the equation by adding or subtracting all the monomials on a selected side of the equation.

To simplify an equation, you have combined like variables by adding or subtracting terms with the same variable and exponents.

What are polynomials?

Polynomials are mathematical expressions that contain multiple terms. Basic operations such as addition, subtraction, multiplication, and division can be used to combine or simplify polynomials.

Adding and subtracting polynomials involves combining like terms, which are those with the same variables and exponents.

Steps to add or subtract polynomial

Adding Polynomials:

Step 1: Put the polynomials into standard form

To add or subtract polynomials, the first step is to put both polynomials in their standard form. In the following example, we have accomplished that step:

(-17x^3 + 8x^2 – 20x + 5) + (20x^3 – 4x^2 + 32x – 3)

Step 2: Arrange the like terms in columns and add as usual

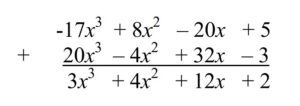
Subtracting Polynomials:

Step 1: Arrange Polynomials

Both polynomials must be arranged in their standard form, which we have accomplished in this example,
(14z3 – 12z2 + 15z – 3) – (7z3 + 3z2 – 10z + 5)
Step 2: Change the sign of subtracting terms and arrange them and subtract

Since the entire second polynomial is subtracted, the sign of each term in the second polynomial can be changed so that all positive terms become negative terms and all negative terms become positive terms. Thus, we have
(14z^3 – 12z^2 + 15z – 3)- 7z^3 – 3z^2 + 10z – 5

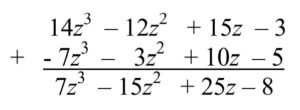
Thus, the result is 7z^3 – 15z^2 + 25z – 8.

FAQ:

What is the purpose of adding, subtracting, dividing, and multiplying polynomials?

You can solve system of equations and cancel unknown variable in the process to find another unknown variable