What Is A Square Number? Many students feel discouraged when thinking about mathematics or solving any problem quickly. Today, I will share a special method for 1 to 100 squares and cubes you wish you knew before, to motivate and find some fun and easy way that will help you save time and get the correct answers.
I will also provide squares and cubes of numbers 1 to 100 in form of a List, Chart, or table. I am going to show you a straightforward strategy that anyone can apply. This strategy will help each student to solve any example quickly and correctly. It is a quick and easy process that requires a few steps so keep reading to find out what they are.
| No. | Square | No. | Square | No. | Square | No. | Square | No. | Square | No. | Square | No. | Square | No. | Square | No. | Square | No. | Square |
| 1 | 1 | 11 | 121 | 21 | 441 | 31 | 961 | 41 | 1681 | 51 | 2601 | 61 | 3721 | 71 | 5041 | 81 | 6561 | 91 | 8281 |
| 2 | 4 | 12 | 144 | 22 | 484 | 32 | 1024 | 42 | 1764 | 52 | 2704 | 62 | 3844 | 72 | 5184 | 82 | 6724 | 92 | 8464 |
| 3 | 9 | 13 | 169 | 23 | 529 | 33 | 1089 | 43 | 1849 | 53 | 2809 | 63 | 3969 | 73 | 5329 | 83 | 6889 | 93 | 8649 |
| 4 | 16 | 14 | 196 | 24 | 576 | 34 | 1156 | 44 | 1936 | 54 | 2916 | 64 | 4096 | 74 | 5476 | 84 | 7056 | 94 | 8836 |
| 5 | 25 | 15 | 225 | 25 | 625 | 35 | 1225 | 45 | 2025 | 55 | 3025 | 65 | 4225 | 75 | 5625 | 85 | 7225 | 95 | 9025 |
| 6 | 36 | 16 | 256 | 26 | 676 | 36 | 1296 | 46 | 2116 | 56 | 3136 | 66 | 4356 | 76 | 5776 | 86 | 7396 | 96 | 9216 |
| 7 | 49 | 17 | 289 | 27 | 729 | 37 | 1369 | 47 | 2209 | 57 | 3249 | 67 | 4489 | 77 | 5929 | 87 | 7569 | 97 | 9409 |
| 8 | 64 | 18 | 324 | 28 | 784 | 38 | 1444 | 48 | 2304 | 58 | 3364 | 68 | 4624 | 78 | 6084 | 88 | 7744 | 98 | 9604 |
| 9 | 81 | 19 | 381 | 29 | 841 | 39 | 1521 | 49 | 2401 | 59 | 3481 | 69 | 4761 | 79 | 6241 | 89 | 7921 | 99 | 9801 |
| 10 | 100 | 20 | 400 | 30 | 900 | 40 | 1600 | 50 | 2500 | 60 | 3600 | 70 | 4900 | 80 | 6400 | 90 | 8100 | 100 | 10000 |
What is a square number? How to find 1 to 100 squares and cubes of any number
A number multiplied with itself is called square number. Finding the Square of a Number is a simple method. We need to bear the given number by itself to find its square number. The square term is always represented by a number raised to the power of 2. For example, the square of 13 is 13 multiplied by 13, i.e., 13×13 = 132 = 169. But as the number gets larger, multiplication becomes time-consuming. In this blog, I will show the simpler method to square a number. For example, Squaring the number 13
1.Square number: Square the last digit of the number
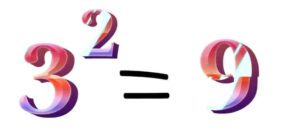
Last digit of the number = 3.
Square of 3 = 3 x 3 = 09
Writing the square of the number from right to left. So write down 9 and carry = 0
2. Double the multiplication of the first and last digit

Here we are trying to square the number 13
Digits of this number are 1 and 3
Twice the multiplication of digits becomes, 2 x 1 x 3 = 6
6 + carry from step 1 (i.e. 0) = 6 + 0 = 06
So the number becomes 69
3. Square the first digit

First digit = 1
Square of number + carry = 1 + 0 0
So the final number becomes 169
If you would like to have more examples or detailed explanations click here
How To Find Cube Of Any Number

1. Cube the last digit of a number

For example, let’s consider the number 12
The last digit of a number is 2
Cube of 2 = 2 x 2 x 2 = 8
Carryover = 0
2: Multiply the digits with each other and then multiply them by 3
Here digits are 1 and 2
therefore 1 x 2 = 2
Thrice the multiplication = 3 x 1 x 2 =6
2.1 Take the last digit and multiply it with the result obtained in the above step
Last digit = 2
Result = 6
Multiplication = 6 x 2 = 12
Carry over from step 1 = 0
therefore 12 + 0 = 12
Write down the last digit and the remaining will now be taken as carrying over.
So the number becomes 28 with a carryover of 1
2.2 Now instead of the last digit take the remaining digit and repeat the same process from step 2.1
Remaining digit = 1
Multiplication = 6 x 1 = 6
Carryover from step 2.1 = 1
therefore 6 + 1 = 7
The number becomes 728 with a carryover of 0
3: Now take the cube of left most digit
Left most digit = 1
Cube of 1 = 1 x 1 x 1 =1
The final number becomes 1728
Conclusion:
What Is A Square Number?: Number multiplied by itself is called a square number. If we go step by step and understand the process, it is easier to find the squares and cubes in less time than actual multiplication.
Summarizing steps for squaring number
1. Cube last digit
2. 3 x (first digit) x (last digit) x (last digit)
3. 3 x (first digit) x (last digit) x (first digit)
4. Cube of the first digit

![Read more about the article Multiplying Binomials – 3 Ways To Solve[pdf]](https://hackonmath.com/wp-content/uploads/2023/01/https___hackonmath.com-2-232x300.png)
Pingback: The Metric System - Math Hacks
Pingback: Arithmetic Progression - Math Hacks
Pingback: How To Study For Maths Exam? - ClusterBooks - The Books Marketplace
Pingback: Resolutions For School Students In 2023 - Math Hacks